Connection between partition functions

1
Connection between partition functions
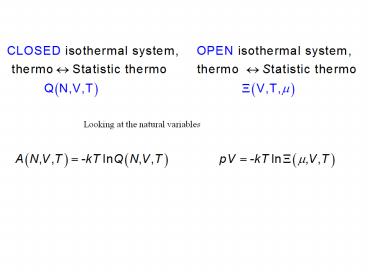
Looking at the natural variables
2
From Q to X
3
Equivalency of ensembles
An example on the equivalency among ensembles
N distinguishable particles, 2 possible states
(DEe with E10) ? aja1,a2,aN where aj 0,
or 1 and therefore
Microcanonical ensemble degeneracy of the mth
level ? number of ways to distribute m objects in
a pool of N (i.e. distribute m quanta to obtain E
total energy)
4
Equivalency of ensembles II
5
Summary of Ensembles
Ensemble Constants Fundamental thermodynamics Total differentials
Microcanonical N,V,E SkblnW
Canonical N,V,T A-kbTlnQ(N,V,T)
Grand-canonical m,V,T pVkbTlnX(m,V,T)
Isothermal-isobaric N,p,T G-kbTlnD(N,p,T)
Useful ensembles ?at least one extensive variable
N,V,or E Generalized ensemble with only
intensive properties, (m,p,T) but
kbTlnZ(m,p,T)0 ? no fundamental function
6
Fluctuations
Fluctuationspontaneous deviation of a mechanical
variable from its mean How much it deviates?
Ergodic hypothesis lttimegt ? ltensemblegt
rms fluctuation of XX(t) is equivalent to sX
7
Fluctuations in Canonical Ensemble
What are the fluctuations in the canonical
ensemble?
8
Fluctuations in Canonical Ensemble II
The spread of the fluctuations corresponds to the
rate at which the energy changes with T
For an ideal gas,
Distribution of energies is like a delta function
centered at ltEgt
9
Fluctuations in Grand canonical
10
Isothermal compressibility
Isothermal compressibility
11
Fluctuations in N
For a canonical ensemble, even thought there are
fluctuations, The energy is distributed
uniformly. Each system is most likely to be found
with energy ltEgt ?canonical ensemble equivalent to
microcanonical (where E is constant)
Fluctuations in N show that a grand canonical
ensemble is most likely to be found with ltNgt
particles ? grand canonical canonical ensemble
equivalent to canonical (where N is constant)
12
P(E)
What is the probability of finding a particular
value of E? P(E)
13
How do we count states
Lets count
14
Distinguishable particles
Canonical ensemble of DISTIGUISHABLE
particles/quasi-particles a,b,n.
15
Example for distinguishable particles
Imagine a system with N1000 degrees of
freedom (1000 quasi particles)
Each particle can be in one of 5 microstates
? There are 51000 states to be sampled (and
counted!!!)
Using the factorization due to equal-but-distingui
shable particles, we only need to enumerate 5
states to evaluate q ? conversion of one N-body
problem to N, 1-body problems
16
Indistinguishable particles
If the particles are INDISTINGUISHABLE
FERMIONS All indices j k,, l must be
different. Hence summations over indices depend
on each other.
BOSONS Indices jkl need not all be
different. Permutations like j kl and kjl
refer to identical states and must occur only
once in the summation.
17
Boltzman Statistics
INDISTINGUISHABLE particles ?
A particular (and common) case T?,d?? number of
available energy states gtgt N
? each an every particle is in a different state
Boltzman Statistics
we have to consider those distribution that are
equivalent, that is
There are N! of these combinations which can be
subtracted from the pool of microstates by
dividing by N!
18
Singel particle energy from Bolztman
Boltzman? number of 1-particle state gtgtnumber of
particles
How many 1-particle states? Remember the sphere
used to explain degeneracy?
number of 1-particle states with an energy lower
than e number of lattice points enclosed by
the sphere in the positive octant