Cardinals

1
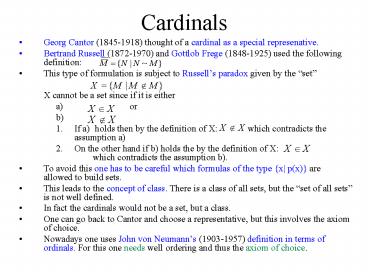
Cardinals

• Georg Cantor (1845-1918) thought of a cardinal as
  a special represenative.
• Bertrand Russell (1872-1970) and Gottlob Frege
  (1848-1925) used the following definition
• This type of formulation is subject to Russells
  paradox given by the set
• X cannot be a set since if it is either
• or
• If a) holds then by the definition of X
  which contradicts the assumption a)
• On the other hand if b) holds the by the
  definition of X which contradicts the
  assumption b).
• To avoid this one has to be careful which
  formulas of the type x p(x) are allowed to
  build sets.
• This leads to the concept of class. There is a
  class of all sets, but the set of all sets is
  not well defined.
• In fact the cardinals would not be a set, but a
  class.
• One can go back to Cantor and choose a
  representative, but this involves the axiom of
  choice.
• Nowadays one uses John von Neumanns (1903-1957)
  definition in terms of ordinals. For this one
  needs well ordering and thus the axiom of choice.

2
Orders of Sets

• A set S is called partially ordered if there
  exists a relation r (usually denoted by the
  symbol ) between S and itself such that the
  following conditions are satisfied
• reflexive a a for any element a in S
• transitive if a b and b c then a c
• antisymmetric if a b and b a then a b
• A set S is called ordered if it is partially
  ordered and every pair of elements x and y from
  the set S can be compared with each other via the
  partial ordering relation.
• A set S is called well-ordered if it is an
  ordered set for which every non-empty subset
  contains a smallest element.

3
Ordinals

• Consider pair (S,ltS) of a set S and a
  well-ordering ltS on S.
• We call (S,ltS) and (T,ltT) equivalent
• (S,ltS)(T,ltT)
• if there is a 1-1 correspondence, which
  preserves the orders, i.e. a bijection fS ?T,
  s.t.
• altb ltgt f(a)ltf(b)
• According to Cantor, the equivalence classes of
  this equivalence relation are ordinals, abstract
  from the nature of the elements to obtain the
  order type.

• Ordinals can be constructed, from the ZF system.
• Von Neumann (1903-1957) constructs ordinals as
  special types of sets, i.e. representatives
• A set S is an ordinal if and only if S is
  totally ordered with respect to set containment
  and every element of S is also a subset of S.
• Call (S,ltS)lt(T,ltT) if and only if S is order
  isomorphic to an initial segment T1 of T, i.e.
  there is a k in T such that ST1aaltk

Ordinals themselves are well-ordered with respect
to the order induced by lt
4
Orders on Ordinals and Cardinals

• Questions
• Can every set be well-ordered?
• Yes (Zermelo), if one assumes the axiom of
  choice.
• Is there an order for ordinals and cardinals?
• This is the case for the ordinals and cardinals
  of finite sets.
• Ordinals can be ordered.
• Cardinals can be ordered if all sets can be
  well-ordered which is equivalent (Zermelo) to the
  axiom of choice.

5
Arithmetic of Ordinals and the sequence

• Let w the ordinal of N in its natural order.
• To add two ordinals A(A,ltA) and B(B,ltB) in the
  following way
• AB(AUB,ltAUB)
• where x ltAUBy if either
• x,y ? A and xltAy
• x,y ? B and xltBy
• x ? A and y ? B
• Caveat is not commutative
• 3ww
• w3gtw
• Ordinals form a ascending sequence
• 1,2,,w, w1, w2,, ww,ww1,

• Now to each ordinal, we can associate its
  cardinal. E.g. the cardinal of w is . This is
  also the cardinal for w1, ww
• Going along the sequence of ordinals, we will
  however discover more cardinals, one after the
  other. In this way let be the first new
  cardinal after and denote the sequence of
  cardinals obtained in this way by
• Zermelo showed that assuming the axiom of choice
  every set can be well ordered.
• Thus assuming the axiom of choice all cardinals
  are among the and the cardinals are well
  ordered.